What Is A Vertical Shift

Learning Outcomes

Graph functions using vertical and horizontal shifts.

Ane kind of transformation involves shifting the entire graph of a part up, downwards, right, or left. The simplest shift is a vertical shift, moving the graph upwards or down, because this transformation involves calculation a positive or negative constant to the function. In other words, we add the same abiding to the output value of the part regardless of the input. For a part [latex]g\left(10\right)=f\left(x\right)+g[/latex], the function [latex]f\left(x\right)[/latex] is shifted vertically [latex]k[/latex] units.

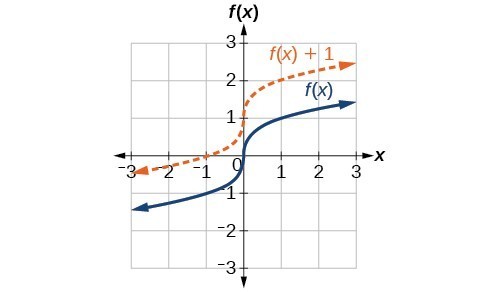
Graph of f of x equals the cubed root of x shifted upward one unit, the resulting graph passes through the point (0,1) instead of (0,0), (1, 2) instead of (1,1) and (-1, 0) instead of (-1, -1)

Vertical shift past [latex]k=1[/latex] of the cube root function [latex]f\left(x\right)=\sqrt[3]{x}[/latex].

To aid you visualize the concept of a vertical shift, consider that [latex]y=f\left(ten\right)[/latex]. Therefore, [latex]f\left(x\correct)+g[/latex] is equivalent to [latex]y+grand[/latex]. Every unit of [latex]y[/latex] is replaced by [latex]y+grand[/latex], so the [latex]y\text{-}[/latex] value increases or decreases depending on the value of [latex]1000[/latex]. The result is a shift upward or down.

A General Note: Vertical Shift

Given a role [latex]f\left(x\right)[/latex], a new function [latex]g\left(x\right)=f\left(x\right)+g[/latex], where [latex]g[/latex] is a abiding, is a vertical shift of the function [latex]f\left(x\correct)[/latex]. All the output values change by [latex]k[/latex] units. If [latex]one thousand[/latex] is positive, the graph will shift upwardly. If [latex]k[/latex] is negative, the graph volition shift down.

Case: Calculation a Constant to a Function

To regulate temperature in a dark-green building, airflow vents near the roof open up and close throughout the 24-hour interval. Effigy 2 shows the area of open up vents [latex]5[/latex] (in square feet) throughout the twenty-four hours in hours after midnight, [latex]t[/latex]. During the summertime, the facilities director decides to try to better regulate temperature by increasing the corporeality of open vents by twenty square feet throughout the day and nighttime. Sketch a graph of this new function.

How To: Given a tabular function, create a new row to represent a vertical shift.

Identify the output row or column.
Determine the magnitude of the shift.
Add the shift to the value in each output cell. Add a positive value for upward or a negative value for downwardly.

Example: Shifting a Tabular Part Vertically

A function [latex]f\left(x\right)[/latex] is given below. Create a table for the part [latex]one thousand\left(x\right)=f\left(x\right)-3[/latex].

[latex]ten[/latex]	2	4	six	eight
[latex]f\left(ten\correct)[/latex]	i	3	vii	eleven

Attempt It

The role [latex]h\left(t\right)=-4.9{t}^{two}+30t[/latex] gives the height [latex]h[/latex] of a ball (in meters) thrown upward from the basis afterward [latex]t[/latex] seconds. Suppose the ball was instead thrown from the tiptop of a 10-thou building. Chronicle this new acme role [latex]b\left(t\right)[/latex] to [latex]h\left(t\right)[/latex], and and then notice a formula for [latex]b\left(t\correct)[/latex].

Show Solution

[latex]b\left(t\correct)=h\left(t\correct)+ten=-four.9{t}^{ii}+30t+10[/latex]

Identifying Horizontal Shifts

We just saw that the vertical shift is a change to the output, or outside, of the role. We will at present await at how changes to input, on the inside of the function, change its graph and significant. A shift to the input results in a movement of the graph of the function left or right in what is known as a horizontal shift.

Graph of f of x equals the cubed root of x shifted left one unit, the resulting graph passes through the point (0,-1) instead of (0,0), (0, 1) instead of (1,1) and (-2, -1) instead of (-1, -1)

Horizontal shift of the function [latex]f\left(x\right)=\sqrt[3]{ten}[/latex]. Annotation that [latex]h=+i[/latex] shifts the graph to the left, that is, towards negative values of [latex]ten[/latex].

For example, if [latex]f\left(x\right)={ten}^{2}[/latex], then [latex]thousand\left(x\right)={\left(x - 2\right)}^{2}[/latex] is a new function. Each input is reduced by 2 prior to squaring the role. The outcome is that the graph is shifted 2 units to the right, considering we would need to increment the prior input by 2 units to yield the same output value as given in [latex]f[/latex].

A General Annotation: Horizontal Shift

Given a role [latex]f[/latex], a new function [latex]m\left(x\right)=f\left(x-h\right)[/latex], where [latex]h[/latex] is a constant, is a horizontal shift of the role [latex]f[/latex]. If [latex]h[/latex] is positive, the graph volition shift right. If [latex]h[/latex] is negative, the graph will shift left.

Example: Adding a Constant to an Input

Returning to our building airflow instance from Instance 2, suppose that in autumn the facilities manager decides that the original venting program starts too late, and wants to begin the entire venting program 2 hours earlier. Sketch a graph of the new office.

How To: Given a tabular office, create a new row to represent a horizontal shift.

Place the input row or column.
Determine the magnitude of the shift.
Add the shift to the value in each input cell.

Case: Shifting a Tabular Function Horizontally

A function [latex]f\left(x\right)[/latex] is given below. Create a table for the function [latex]one thousand\left(x\right)=f\left(x - iii\right)[/latex].

[latex]x[/latex]	2	iv	vi	eight
[latex]f\left(x\right)[/latex]	1	3	7	11

Example: Identifying a Horizontal Shift of a Toolkit Function

This graph represents a transformation of the toolkit role [latex]f\left(x\correct)={x}^{2}[/latex]. Relate this new office [latex]g\left(10\right)[/latex] to [latex]f\left(10\correct)[/latex], and and then detect a formula for [latex]g\left(x\correct)[/latex].

Graph of a parabola.

Example: Interpreting Horizontal versus Vertical Shifts

The function [latex]Yard\left(k\right)[/latex] gives the number of gallons of gas required to drive [latex]one thousand[/latex] miles. Interpret [latex]K\left(one thousand\correct)+10[/latex] and [latex]G\left(thousand+x\right)[/latex].

Effort Information technology

Given the function [latex]f\left(ten\correct)=\sqrt{ten}[/latex], graph the original function [latex]f\left(ten\right)[/latex] and the transformation [latex]thou\left(ten\correct)=f\left(x+ii\right)[/latex] on the same axes. Is this a horizontal or a vertical shift? Which way is the graph shifted and past how many units?

Evidence Solution

A horizontal shift results when a abiding is added to or subtracted from the input. A vertical shift results when a constant is added to or subtracted from the output.

Endeavor It

Desmos can graph transformations using function note. Use an online graphing tool to graph the toolkit function [latex]f(x) = x^two[/latex]
At present, enter [latex]f(10+five)[/latex], and [latex]f(x)+5[/latex] in the next two lines.

Now have Desmos make a table of values for the original part. Include integer values on the interval [latex][-5,five][/latex]. Replace the column labeled [latex]y_{1}[/latex] with [latex]f(x_{ane})[/latex].

At present replace [latex]f(x_{1})[/latex] with [latex]f(x_{one}+3)[/latex], and [latex]f(x_{1})+three[/latex].

What are the corresponding functions associated with the transformations you have graphed?

Contribute!

Did you have an idea for improving this content? We'd beloved your input.

Meliorate this pageLearn More